3. DM HALO SCALING LAWS

The correlations between halo r_c, ₀, and and galaxy absolute magnitude M_B are illustrated in Figures 2 - 4. Figure 2 shows results derived using isothermal halos. Figure 3 shows results derived using pseudo-isothermal halos. Figure 4 combines all of the data, as discussed below.

Figure 2. Dark matter parameter correlations derived using isothermal halos in rotation curve decompositions of Sc - Im galaxies (circles). Central dark matter densities of dSph galaxies (filled triangles) and dI galaxies (upside-down filled triangles) are derived via King (1966) model fits; corresponding rc and values are for the stars (open triangles). The lines are least-squares fits to the Sc-Im galaxies omitting NGC 4605 (open circle). Parameter sources: ABP; Blais-Ouellette et al. (1999); Bosma et al. (2003); Carignan & Puche (1990); Carignan & Purton (1998); Carignan et al. (1988); Côté et al. (2000); Jobin & Carignan (1990); Martimbeau et al. (1994); Meurer et al. 1996); Puche et al. (1991); Sicotte & Carignan (1997); Verdes-Montenegro et al. (1997).

The scatter about the correlations is slightly smaller in Figure 2 than in Figure 3. It is not surprising that decompositions based on the isothermal sphere are better behaved, given the slow rise of the PITS rotation curve to its asymptotic value (Fig. 1). However, rotation curve decompositions based on isothermal and PITS models separately give essentially the same correlations.

Figure 3. Dark matter parameter correlations derived using the pseudo-isothermal sphere to model DM halos in rotation curve decompositions of Sc - Im galaxies (filled squares). The straight lines are least-squares fits to all the points. Parameter sources: Broeils (1992); Corbelli (2003); de Blok & McGaugh (1996, 1997); de Blok, McGaugh, & Rubin (2001); Meurer et al. (1996); Meurer, Staveley-Smith, & Killeen (1998); Miller & Rubin (1995); Skillman et al. (1987); Swaters, Madore, & Trewhella (2000); Swaters et al. (2003); van Zee et al. (1997); Verheijen (1997); Weldrake, de Blok, & Walter (2003).

There are 31 galaxies with isothermal DM decompositions and 37 with PITS decompositions. Only 13 galaxies are common to both samples. So the results in Figures 2 and 3 are largely independent. Clearly, we want to combine the two samples. We do this by scaling the correlations in Figure 3 to those in Figure 2.

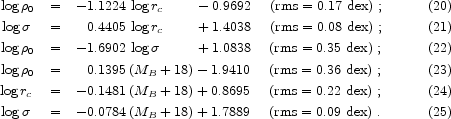
Least-squares fits to the correlations in Figure 2 (omitting NGC 4605) give:

Least-squares fits to the correlations in Figure 3 give:

The two samples have essentially the same average absolute magnitude: < M_B > = - 18.12 for objects analyzed with isothermals and < M_B > = - 17.83 for those analyzed with pseudo-isothermals. The average of the above values is M_B = - 17.97. Requiring that the correlations agree at M_B = - 18 provides the scaling of the PITS results to those measured with the isothermal sphere (ITS):

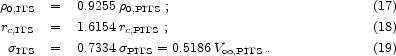Here we explicitly identify the parameters derived with the different DM models. This scaling is intermediate between the middle and right panels of Figure 1.

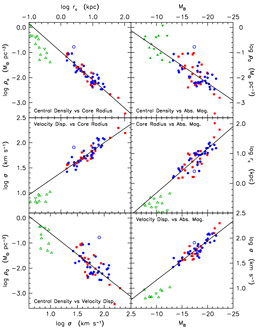Figure 4. Dark matter parameter correlations for Sc-Im, dwarf spheroidal (dSph), and dI galaxies. The symbols are the same as in Figures 2 and 3. For dSph and dI galaxies, 0 is a meaure of the DM but rc and are visible-matter parameters. The straight lines are least-squares fits to the Sc - Im galaxies omitting NGC 4605 (open circle). NGC 4605 deviates from the correlations because the mass-to-light ratio of the disk was reduced significantly from its maximum disk value to kill off one-armed spiral instabilities (see ABP). It is the only such galaxy retained in the sample. In Figures 2 - 4, distances are based on a Virgocentric flow field calibrated using Cepheids and surface brightness fluctuations. They correspond to H0 70 km s-1 Mpc-1.

Figure 4 shows the combined sample after scaling of the pseudo-isothermal results to those determined with isothermal halos. Based on 55 galaxies spanning 9 magnitudes in M_B, the correlations are quite robust. Uncertainties in rotation curve decomposition are unlikely to threaten them, although the slopes are still uncertain. If we keep only the galaxies with the most leverage on the DM, say the 11 galaxies whose rotation curves reach out to at least 10 times the scale length of the disk (one of these is NGC 3198, in which the ratio is 11.4), then we get essentially the same correlations with essentially the same scatter. Least-squares fits to the points in Figure 4 give:

In physically more transparent terms (10⁹ L_B is M_B = - 17.03),

Equations 26 and 27 imply that halo surface density, which is proportional to ₀ r_c, is nearly independent of galaxy luminosity. This near-independence is illustrated explicitly in Figure 5. It implies a Tully-Fisher (1977) relation similar to equation 29 (cf. Fall 2002). Since mass M ₀ r_c³ and since ₀ r_c 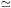constant, M^1/2 r_c. Then M ² r_c implies that M ⁴. We find that L_B ^5.1. This suggests that M / L_B L_B^-0.22. If we correct for the slight dependence of halo surface density on L_B (Fig. 5), then this becomes M / L_B L_B^-0.24. Slope uncertainties are significant, so we should be cautious in how much we interpret this result. However, it is a plausible estimate of the degree to which small galaxies are more DM dominated than large ones. UGC 2885 (M_B = - 22.3) is brighter than UMi (M_B = - 7.6) by 14.7 mag. Over 15 mag, M / L_B L_B^-0.24 implies that M / L_B changes by a factor of 26. This is entirely reasonable.

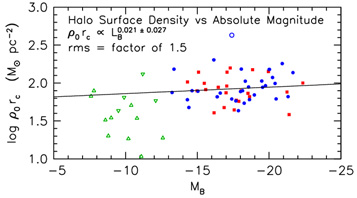Figure 5. Logarithm of 0 rc, which is proportional to the projected surface density of DM halos, as a function of absolute magnitude. The symbols are the same as in Figures 2 - 4. The straight line (key) is a least-squares fit to the Sc - Im galaxies omitting NGC 4605. Within the errors, the surface densities of DM halos of late-type galaxies are independent of galaxy luminosity.