2. BIG BANG NUCLEOSYNTHESIS AND THE PRIMORDIAL ABUNDANCES

Since the early universe is hot and dense, interactions among the various particles present are rapid and equilibrium among them is established quickly. But, as the universe expands and cools, there are departures from equilibrium; these are at the core of the most interesting themes of our story.

2.1. An Early Universe Chronology

At temperatures above a few MeV, when the universe is tens of milliseconds old, interactions among photons, neutrinos, electrons, and positrons establish and maintain equilibrium (T = T = T_e). When the temperature drops below a few MeV the weakly interacting neutrinos decouple, continuing to cool and dilute along with the expansion of the universe (T a^-1, n T³, and T⁴).

2.1.1. Neutron - Proton Interconversion

Up to now we haven't considered the baryon (nucleon) content of the universe. At these early times there are neutrons and protons present whose relative abundance is determined by the usual weak interactions.

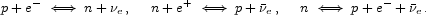(24)

As time goes by and the universe cools, the lighter protons are favored over the heavier neutrons and the neutron-to-proton ratio decreases, initially as n / p exp (- m / T), where m = 1.29 MeV is the neutron-proton mass difference. As the temperature drops below roughly 0.8 MeV, when the universe is roughly one second old, the rate of the two-body collisions in eq. 24 becomes slow compared to the universal expansion rate and deviations from equilibrium occur. This is often referred to as "freeze-out", but it should be noted that the n / p ratio continues to decrease as the universe expands, albeit at a slower rate than if the ratio tracked the exponential. Later, when the universe is several hundred seconds old, a time comparable to the neutron lifetime (_n = 885.7 ± 0.8 sec.), the n / p ratio resumes falling exponentially: n / p exp(- t / _n). Notice that the n/p ratio at BBN depends on the competition between the weak interaction rates and the early universe expansion rate so that any deviations from the standard model (e.g. 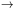+ _X) will change the relative numbers of neutrons and protons available for building more complex nuclides.

2.1.2. Building The Elements

At the same time that neutrons and protons are interconverting, they are also colliding among themselves to create deuterons: n + p 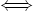D + . However, at early times when the density and average energy of the CMB photons is very high, the newly-formed deuterons find themselves bathed in a background of high energy gamma rays capable of photodissociating them. As we shall soon see, there are more than a billion photons for every nucleon in the universe so that before a neutron or a proton can be added to D to begin building the heavier nuclides, the D is photodissociated. This bottleneck to BBN beginning in earnest persists until the temperature drops sufficiently so that there are too few photons energetic enough to photodissociate the deuterons before they can capture nucleons to launch BBN. This occurs after e^± annihilation, when the universe is a few minutes old and the temperature has dropped below 80 keV (0.08 MeV).

Once BBN begins in earnest, neutrons and protons quickly combine to form D, ³H, ³He, and ⁴He. Here, there is another, different kind of bottleneck. There is a gap at mass-5; there is no stable mass-5 nuclide. To jump the gap requires ⁴He reactions with D or ³H or ³He, all of which are positively charged. The coulomb repulsion among these colliding nuclei suppresses the reaction rate ensuring that virtually all of the neutrons available for BBN are incorporated in ⁴He (the most tightly bound of the light nuclides), and also that the abundances of the heavier nuclides are severely depressed below that of ⁴He (and even of D and ³He). Recall that ³H is unstable and will decay to ³He. The few reactions which manage to bridge the mass-5 gap mainly lead to mass-7 (⁷Li, or ⁷Be which later, when the universe has cooled further, will capture an electron and decay to ⁷Li); the abundance of ⁶Li is below that of the more tightly bound ⁷Li by one to two orders of magnitude. There is another gap at mass-8. This absence of any stable mass-8 nuclides ensures there will be no astrophysically interesting production of heavier nuclides.

The primordial nuclear reactor is short-lived, quickly encountering an energy crisis. Because of the falling temperatures and the coulomb barriers, nuclear reactions cease rather abruptly when the temperature drops below roughly 30 keV, when the universe is about 20 minutes old. As a result there is "nuclear freeze-out" since no already existing nuclides are destroyed (except for those that are unstable and decay) and no new nuclides are created. In ~ 1000 seconds BBN has run its course.