10. REIONIZATION

When an isolated point source of ionizing radiation turns on in a neutral medium, the ionized volume initially grows in size at a rate fixed by the emission of UV photons, and an ionization front separating the H II and H I regions propagates into the neutral gas. Most photons travel freely in the ionized bubble, and are absorbed in a transition layer. The evolution of an expanding H II region is governed by the equation

(8)

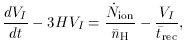
where V_I is the proper volume of the ionized zone, 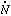_ion is the number of ionizing photons emitted by the central source per unit time, 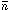_H is the mean hydrogen density of the expanding IGM, H is the Hubble constant, and 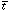_rec is the hydrogen mean recombination timescale,

(9)

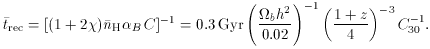
One should point out that the use of a volume-averaged clumping factor, C < n_HII² > / 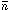_HII², in the recombination timescale is only justified when the size of the H II region is large compared to the scale of the clumping, so that the effect of many clumps (filaments) within the ionized volume can be averaged over. The validity of this approximation can be tested by numerical simulations (see Figure 7). Across the I-front the degree of ionization changes sharply on a distance of the order of the mean free path of an ionizing photon. When 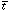_rec is much smaller than the Hubble time, the growth of the H II region is slowed down by recombinations in the highly inhomogeneous medium, and its evolution can be decoupled from the expansion of the universe.

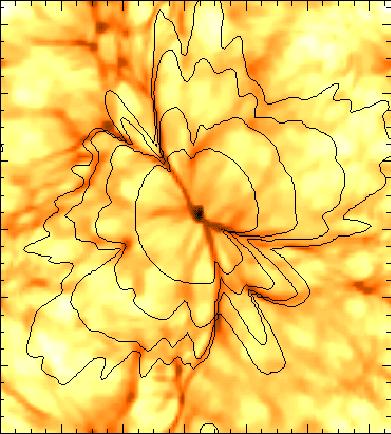
Figure 7. Simulating the reionization of the universe: propagation of an ionization front in a 1283 cosmological density field. A ``mini-quasar'' with = 5 x 1053 s-1 was turned on at the densest cell, in a virialized halo of total mass 1.3 x 1011 M. The box length is 2.4 comoving Mpc. The solid contours give the position of the front at 0.15, 0.25, 0.38, and 0.57 Myr after the quasar has switched on at z = 7. The underlying greyscale image indicates the initial H I density field. (From [1].)

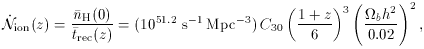
which is independent of the (unknown) previous emission history of the universe: only rates above this value will provide enough UV photons to ionize the IGM by that epoch. One can then compare our estimate of 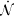_ion to the inferred contribution from QSOs and star-forming galaxies. The uncertainty on this critical rate is difficult to estimate, as it depends on the clumpiness of the IGM (scaled in the expression above to the value inferred at z = 5 from numerical simulations [20]) and the nucleosynthesis constrained baryon density. The evolution of the critical rate as a function of redshift is plotted in Figure 6 (right). While 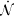_ion is comparable to the quasar contribution at z 3, there is some indication of a deficit of Lyman-continuum photons at z = 5. For bright, massive galaxies to produce enough UV radiation at z = 5, their space density would have to be comparable to the one observed at z 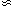3, with most ionizing photons being able to escape freely from the regions of star formation into the IGM. This scenario may be in conflict with direct observations of local starbursts below the Lyman limit showing that at most a few percent of the stellar ionizing radiation produced by these luminous sources actually escapes into the IGM [31]. ⁽⁵⁾

It is interesting to convert the derived value of 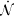_ion into a ``minimum'' star formation rate per unit (comoving) volume, _*:

(11)

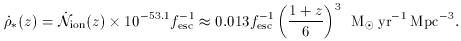
The star formation density given in the equation above is comparable with the value directly ``observed'' (i.e., uncorrected for dust reddening) at z 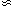3 [37]. The conversion factor assumes a Salpeter IMF with solar metallicity, and has been computed using a population synthesis code [4]. It can be understood by noting that, for each 1 M of stars formed, 8% goes into massive stars with M > 20 M that dominate the Lyman-continuum luminosity of a stellar population. At the end of the C-burning phase, roughly half of the initial mass is converted into helium and carbon, with a mass fraction released as radiation of 0.007. About 25% of the energy radiated away goes into ionizing photons of mean energy 20 eV. For each 1 M of stars formed every year, we then expect

(12)

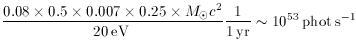
to be emitted shortward of 1 ryd.