The techniques discussed in Section 8 have been used to search for the thermal and kinematic Sunyaev-Zel'dovich effects towards a large number of clusters, and the non-thermal Sunyaev-Zel'dovich effects towards a few radio galaxies. Over the past few years this work has been increasingly successful, because of the high sensitivity that is now being achieved, and the careful controls on systematic errors that are used by all groups. The most impressive results are those obtained from radio interferometers, which are producing images of the cluster Sunyaev-Zel'dovich effects that can be compared directly with images of cluster X-ray structures. In the present section I collect all published results on Sunyaev-Zel'dovich effects of which I am aware, and review the reliability of the measurements.
Table 4 contains the final
result measured in each series of observations for each of the
clusters that has been observed in the Sunyaev-Zel'dovich effect. Not all
papers in Tables 1,
2, and
3 are represented in
Table 4, since
I have excluded interim reports where they have been superseded by later
work (which often involves improved calibrations and assessments of
systematic errors). The column marked ``O/C'' reports whether the
quoted value of 
TRJ is as observed or as deconvolved, by
the observers, into some central estimated Sunyaev-Zel'dovich effect. As
explained in
Secs 8.2 and 8.3,
model-fitting to produce a
central decrement is commonly used when only a small fraction of the
central decrement can be recorded by the telescope.
The overall set of clusters for which Sunyaev-Zel'dovich effects have been sought does not constitute a well-defined sample in any sense. Early work on the Sunyaev-Zel'dovich effects concentrated on clusters with strong X-ray sources, or for which the radio source contamination was known to be small. Abell 426 (the Perseus cluster) is an example of a cluster observed for the first reason, despite its strong radio sources (Lake & Partridge 1980). Abell 665, on the other hand, was observed principally because it was known to be largely free of strong radio sources, but also because it is the richest cluster in the Abell catalogue (Birkinshaw et al. 1978a). With more sensitive X-ray surveys, X-ray images, and X-ray spectroscopy, several clusters with exceptional X-ray properties have also been observed. Examples are the high-luminosity cluster CL 0016+16 (Birkinshaw et al. 1981a), and the high-temperature cluster Abell 2163 (Holzapfel et al. 1997b).
More recently, there has been some effort to observe complete samples of clusters of galaxies selected on the basis of their X-ray or optical properties, since the interpretation of cluster Sunyaev-Zel'dovich effects in cosmological terms may be biased by the use of the ad hoc samples that have been assembled to date. Initial steps in these directions have been taken by, for example, Myers et al. (1997). At present, though, it is not possible to use the sample of clusters contained in Table 4 to make reliable statistical statements about the effects of clusters on the CMBR. Attempts to normalize a Sunyaev-Zel'dovich effect cluster luminosity function (e.g., Bartlett & Silk 1994a) based on these clusters may not be safe.
Extreme care is needed in interpreting the results given in this
table. First, the datum that is recorded, TRJ, is the
measured Sunyaev-Zel'dovich effect from the cited paper at the most significant
level observed (code O), or the central Sunyaev-Zel'dovich effect in the
cluster,
as fitted based on some model of the cluster gas (code C), and which
would be seen in the Rayleigh-Jeans limit if the cluster were observed
with infinitely good angular resolution. That is, for C codes,
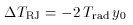
It is not simple to convert from the measured effects to the central
effects, since proper account must be taken of the method used to
observe the cluster and the efficiency factor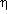 
(see
Fig. 14, for example). For some
observations, for
example with multichannel bolometer systems, it may have been
necessary for the observers to undertake a significant fitting
exercise to extract the central TRJ, TRJ0, with the result
depending on the model of the cluster gas adopted
(Sec. 8.2). Clusters with only poor X-ray images are
therefore difficult to assess, but in cases in which there is good
X-ray data this fitting step is relatively reliable. Thus it can be
shown, for example, that recent results for Abell 2218 are in much
better agreement than is apparent from Table 4 (see
later).
Many of the observations made with bolometers express their results in
terms of y0, the central value of y through the target
cluster. In those cases (e.g.,
Holzapfel et
al. 1997b),
I have converted the results to central decrements using
(93). In cases where the peak beam-averaged
value of TRJ
is stated (e.g.,
Chase et al.
1987),
that value is preferred in the table.
For the interferometric data, the measured flux densities on
the most appropriate (usually lowest-resolution) maps have been
converted into measured brightness temperatures using the synthesized
beamsize quoted. That is, it is assumed that the synthesized beam is
an elliptical Gaussian, with solid angle 
ab (calculated from the full widths to
half-maximum in two directions, h a x
h b), and the brightness temperature is obtained from
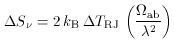
which in convenient units, becomes (95)
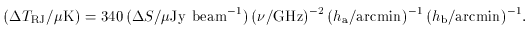
In the case of the Partridge et al. (1987) data, I have estimated the error on the central Sunyaev-Zel'dovich effect from their visibility curves, taking rough account of the systematic errors in the data caused by correlator offsets.
For the radiometric results, which are the bulk of the entries in
Table 4, the values of TRJ are taken
directly from the papers. The results from
Rudnick (1978)
are given
for a 2-arcmin FWHM structure at the cluster center, since this is the
closest match to the resolution of the telescope used. Rudnick also
quotes more sensitive results for TRJ at a number of larger
angular scales by convolving the data. These larger scales may be more
appropriate for some clusters.
In many cases the radiometric data have been adjusted for the effects of cluster and background radio sources. These adjustments are not necessarily consistent between the different papers: as further radio work has been done on the clusters, some have shown that substantial radio source corrections are needed (see, for example, Abell 2507). Sometimes the detections of these radio sources led to the cluster observations being abandoned (e.g., for Abell 426). For other clusters, later work may have used better source corrections and is often more reliable on these grounds alone. Many of the clusters with radiometric Sunyaev-Zel'dovich results reported here have had little supporting work on the radio source environment. This makes it difficult to assess the extent to which the results are affected by radio source contamination.
A number of trends are clear in Table 4. Early observations were dominated by single-dish radiometers (e.g., Birkinshaw et al. 1981b). More recently, the bolometric technique has been used, specially because of the interest in detecting the effect near 190 GHz, where the kinematic effect is more obvious (e.g., Holzapfel et al. 1997b). Finally, the completion of the Ryle array and the use of the OVMMA and BIMA for Sunyaev-Zel'dovich effect measurements has produced a series of sensitive maps of clusters (e.g., Jones et al. 1993; Carlstrom et al. 1996), where some evidence of the cluster structure is seen (e.g., for CL 0016+16; Carlstrom et al. 1996; Sec. 8.3).
Despite the increasing use of these new techniques, single-dish radiometry is still used - principally for survey work, to locate target clusters with significant Sunyaev-Zel'dovich effects that might be the subjects of detailed mapping later. Thus observations at OVRO with the 40-m telescope at present are concentrating on a sample of clusters selected because of their excellent exposures by the ROSAT PSPC. Myers et al. (1997) are making a survey of another sample of clusters with the OVRO 5.5-m telescope.
The results in Table 4 span more than 20 years of work on the Sunyaev-Zel'dovich effect, and involve a number of different techniques with different observing characteristics. Thus it is difficult to compare the results of different groups for any one cluster without taking detailed account of the structure of the cluster and the details of the method used. This causes the apparent disagreements between different groups' results to be accentuated. Nevertheless, there are clusters for which the data (particularly the more recent data) are largely in agreement, and clusters for which the situation is less clear.
| TRJ (mK)
| Reference | Telescope; frequency |
| -1.94 ± 0.54 | Gull & Northover 1976 | Chilbolton 25-m; 10.6 GHz |
| -1.09 ± 0.28 | Birkinshaw et al. 1978a | Chilbolton 25-m; 10.6 GHz |
| -1.49 ± 0.23 | Birkinshaw et al. 1978b | Chilbolton 25-m; 10.6 GHz |
| -1.05 ± 0.21 | Birkinshaw et al. 1981b | Chilbolton 25-m; 10.6 GHz |
| -0.38 ± 0.19 | Birkinshaw & Gull 1984 | OVRO 40-m; 10.7 GHz |
| -0.34 ± 0.05 | Birkinshaw et al. 1984 | OVRO 40-m; 20.3 GHz |
| -0.31 ± 0.13 | Birkinshaw & Gull 1984 | OVRO 40-m; 20.3 GHz |
| -0.39 ± 0.03 | Birkinshaw & Moffet 1986 | OVRO 40-m; 20.3 GHz |
| -0.36 ± 0.10 | Birkinshaw 1986 | OVRO 40-m; 20.3 GHz |
| -0.35 ± 0.09 | Birkinshaw 1990 | OVRO 40-m; 20.3 GHz |
| -0.40 ± 0.05 | Birkinshaw et al. 1998 | OVRO 40-m; 20.3 GHz |
Consider, for example, the cluster Abell 2218, for which a particularly large number of measurements are available. First, consider the history of results for Abell 2218 obtained by the group with which I have been working. The published results from 1976 to 1996 are given in Table 5. These results are not independent: later results from the Chilbolton 25-m telescope included the data used in earlier papers, and the OVRO 40-m results also changed as more data were accumulated, and as the radio source corrections and data calibrations were better understood.
The internal consistency of the early data is clearly poor. The final result based on the Chilbolton data is only marginally consistent with the first published result, suggesting that the later data were quite inconsistent with the earlier data. Since a number of changes in the configuration of the Chilbolton system occurred during the period that data were taken, it is likely that this inconsistency arose from unrecognized systematic errors, possibly involving strong ground signals entering through distant sidelobes.
Later data, from the OVRO 40-m telescope, appear more consistent -
the 10.7-GHz result and the 20.3-GHz results seem to be indicating
that the value of TRJ towards the center of the cluster is about
-0.35 mK. However, the observing characteristics of these
observations was very different, and the low-significant detection at
10.7 GHz is due almost completely to a correction for contaminating
radio sources near the center of the cluster.
If it is assumed that the atmosphere of Abell 2218 follows the model
(64), and is isothermal, then the structural parameters
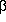= 0.65 ± 0.05
and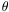 
c = 1.0 ±
0.1 arcmin derived from X-ray observations
(Birkinshaw & Hughes
1994)
may be used to calculate the efficiencies with which the cluster was observed
by any telescope. For observations of the Sunyaev-Zel'dovich effect of
Abell 2218
with the Chilbolton 25-m telescope, the OVRO 40-m
telescope at 10.7 GHz, and the OVRO 40-m telescope at 20.3 GHz, these efficiencies
are about 0.35, 0.49, and 0.60, respectively. The inferred
central Sunyaev-Zel'dovich effects from the cluster according to the
final results
from these three telescope configurations are therefore -3.0 ±
0.6, -0.77 ± 0.38, and -0.67 ± 0.08 mK. The result from the
Chilbolton 25-m telescope is clearly inconsistent
with the other two
measurements. Only a very contrived structure for the cluster
atmosphere could cause such differences and be consistent with the
other Sunyaev-Zel'dovich effect data and the X-ray image and spectrum. Thus an
economical assumption is that the early data were badly contaminated
by systematic errors, and should be discarded, and that the true
central decrement from Abell 2218 is near -0.7 mK.
Another effect that can be seen in Table 5 is the strong variation in the errors quoted for the 20.3-GHz data as a function of time. The smallest error (± 0.03 mK, in Birkinshaw & Moffet 1986) represents the error on the data accumulated at that time if all the data are considered to be drawn from a single, static, Gaussian distribution. The largest error, ± 0.13 mK, in Birkinshaw & Gull (1984), is based on the smallest amount of data, under the same assumptions. On the other hand, the entry for Birkinshaw (1986) is based on substantially more data than in Birkinshaw & Moffet (1986), but includes a generous allocation for possible systematic errors. Later entries in the Table include further data, and were derived with detailed analyses for systematic errors. It should be noted that the final result in the table, -0.40 ± 0.05 mK, contains no contribution from the background CMBR anisotropies, so that the error represents the reproducibility of the measurement rather than the external error that would be achieved if Abell 2218 could be observed against another patch of the background radiation.
Of course, Table 5 illustrates principally the difficulty in measuring the Sunyaev-Zel'dovich effect signals in the presence of systematic errors with unknown characteristics: reductions in the error are principally achieved by stronger controls against systematic errors (for example by observing multiple regions of blank sky, performing checks for radio source contamination, and so on). More rigorous controls against systematic error are obtained by comparing the results from different groups who observe the same cluster in different ways. The most frequently-observed cluster is Abell 2218, and Table 6 lists the central decrements for Abell 2218 deduced from 16 independent measurements using the same model atmosphere as in discussion of Table 5.
| TRJ0 (mK)
| Reference |
| -2.6 ± 1.2 | Perrenod & Lada 1979 |
| +2.2 ± 1.1 | Lake & Partridge 1980 |
| -3.04 ± 0.61 | Birkinshaw et al. 1981b |
| -4.49 ± 0.80 | Schallwich 1982 |
| +0.8 ± 2.4 | Lasenby & Davies 1983 |
| -0.77 ± 0.38 | Birkinshaw & Gull 1984 |
| -0.48 ± 0.39 | Uson 1985 |
| +7.8 ± 5.3 | Radford et al. 1986 |
| +0.21 ± 0.57 | Radford et al. 1986 |
| +0.46 ± 0.36 | Radford et al. 1986 |
| +0.40 ± 0.70 | Partridge et al. 1987 |
| -3.2 ± 1.1 | Klein et al. 1991 |
| -0.90 ± 0.10 | Jones 1995 |
| -0.88 ± 0.26 | Uyaniker et al. 1997 |
| -0.67 ± 0.08 | Birkinshaw 1998 |
| -0.68 ± 0.19 | Tsuboi et al. 1998 |
It is at once apparent from Table 6 that the
individual results are inconsistent: the early data are often
scattered with dispersion
several times their nominal error about the later data. In some of the
early papers, large parasitic signals from ground spillover have been
removed (e.g.,
Perrenod & Lada 1979),
but there
remains a suspicion that residual systematic errors are present in the
data. Overall, the later data are in much better agreement. A notable
exception is the result of
Klein et al.
(1991),
where
the measured decrement is consistent with predictions based on other
data, but its location on the sky is far from the X-ray center of the
cluster so that the implied central decrement in Table 6 is
unrealistically large. If an average is taken over these data, and the most
obviously discordant results are excluded, then the central decrement in
Abell 2218 is found to be -0.74 ± 0.07 mK. The error here has been
increased to take some crude account of the remaining discordance in
the data (the value of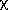 
2
= 15 with 10 degrees of freedom).
| Cluster | Recent measurement | Independent confirmation |
| Abell 478 | Myers et al. 1997 | . . . |
| Abell 665 | Birkinshaw et al. 1998 | Grainge 1996 |
| Abell 697 | Grainge 1996 | . . . |
| Abell 773 | Carlstrom et al. 1996 | Grainge et al. 1993 |
| Abell 990 | Grainge et al. 1996 | . . . |
| Abell 1413 | Grainge et al. 1996 | . . . |
| Abell 1656 | Herbig et al. 1995 | . . . |
| Abell 1689 | Holzapfel et al. 1997b | . . . |
| Abell 2142 | Myers et al. 1997 | . . . |
| Abell 2163 | Holzapfel et al. 1997b | . . . |
| Abell 2218 | Birkinshaw et al. 1998 | Jones 1995 |
| Abell 2256 | Myers et al. 1997 | . . . |
| CL 0016+16 | Carlstrom et al. 1996 | Birkinshaw et al. 1998 |
The Sunyaev-Zel'dovich effect results for Abell 2218 are generally in better
agreement now than they were for the first few years of reported
measurements. This suggests that several groups are now able to
measure reliable Sunyaev-Zel'dovich effects, and based on this
conclusion, I have collected into Table 7 the set of
all Sunyaev-Zel'dovich effects that I believe are both significant (at
> 4
)
and reliable. These objects constitute a set for which a simultaneous
analysis of the Sunyaev-Zel'dovich effect data and the X-ray data may
provide useful constraints on the cluster atmospheres
(Sec. 10), and
possibly a measurement of the Hubble constant
(Sec. 11). Of the thirteen clusters in the
table, seven were first detected using single-dish radiometers,
two using bolometers, and four using interferometers. Only four of
these detections have independent confirmations at significance >
4. Much work remains to be done
to measure the Sunyaev-Zel'dovich effects in
these clusters, and all three measurement techniques still have their
place in Sunyaev-Zel'dovich effect research, although bolometer
measurements are becoming more important, and interferometric maps of
the effect are probably the most reliable.
Detections at lower significance exist for more objects, including the lines of sight towards two high-redshift quasars (PHL 957, Andernach et al. 1986; PC 1643+4631, Jones et al. 1997). These detections may arise from distant clusters of galaxies along the lines of sight, or from the host clusters of the quasars themselves, or from some other cause. However, if the Sunyaev-Zel'dovich effects arise from line-of-sight objects, then observations towards ``blank'' sky regions should show Sunyaev-Zel'dovich effects as often as observations towards the quasars - it is not yet clear whether this is the case, so the interpretation of these Sunyaev-Zel'dovich effects and the limits from observations of other quasars (Jones et al. 1997) or blank fields (Richards et al. 1997) is at present obscure.
Further complications in the interpretation of these results have arisen as deep optical and X-ray followups have been made. Thus for the PC 1643+4631 field, Saunders et al. (1997) find no cluster that might be responsible for a Sunyaev-Zel'dovich effect in deep optical images, and Kneissl, Sunyaev & White (1998) find no X-ray emission associated with hot gas. The interpretation of the CMBR anisotropy as a Sunyaev-Zel'dovich effect has become difficult because of the high redshift needed for a relatively massive cluster that could hold a detectable amount of hot gas (Bartlett et al. 1998). Alternative models involving kinematic effects from colliding QSO winds (Natarayan & Sigurdsson 1997), extreme Rees-Sciama effects, etc. are being considered, but seem implausible. Independent observational confirmation of the reality of these microwave background structures is therefore a priority: early results are yielding a mixed verdict.